< >

Statistical Design of Experiments Statistical Design of Experiments Unit I Unit I Introduction to Statistical Introduction to Statistical Design of Experiments Design of Experiments

2 Statistical Design of Experiments Statistical Design of Experiments Statistical Design of Experiments Statistical Design of Experiments To determine if there is a better way to do To determine if there is a better way to do something something An experiment is performed under controlled An experiment is performed under controlled conditions to determine an unknown effect conditions to determine an unknown effect Statistical Design of Experiments (DOE) is a Statistical Design of Experiments (DOE) is a method of controlling one or more input method of controlling one or more input variables at two or more levels to determine if variables at two or more levels to determine if and how one or more output variables change and how one or more output variables change Why Experiment? Why Experiment?

3 Statistical Design of Experiments Statistical Design of Experiments Optimize Process/Product Optimize Process/Product Performance Performance Minimize Variability/Robust Design Minimize Variability/Robust Design Identify Factors that effect Process Identify Factors that effect Process Performance Performance Minimize Development Time! Minimize Development Time! Statistical Design of Experiments Statistical Design of Experiments

Example:  Suppose you are building a new house and must landscape.  How will you grow the Example:  Suppose you are Example:  Suppose you are building a new house and building a new house and must landscape.  How will must landscape.  How will you grow the you grow the Statistical Design of Experiments Statistical Design of Experiments • Seed Types • • Seed Types Seed Types • Moisture • • Moisture Moisture • Fertilizer • • Fertilizer Fertilizer • Soil • • Soil Soil 4 Statistical Design of Experiments Statistical Design of Experiments

5 Statistical Design of Experiments Statistical Design of Experiments Response Variable Response Variable The observed/measured result The observed/measured result “Output variable, dependent “Output variable, dependent variable” variable” Quality characteristic Quality characteristic Is the “what” to be improved Is the “what” to be improved through Experimentation. through Experimentation. From the Example:  Thick, Green Grass From the Example:  Thick, Green Grass Thick, Green Grass is a Response is a Response Variable Variable

6 Statistical Design of Experiments Statistical Design of Experiments Main Effects Main Effects Independent Variable/Input Factor Independent Variable/Input Factor -- -- an an assignable cause which may affect the assignable cause which may affect the response variable and which is included response variable and which is included at different levels in the experiment. at different levels in the experiment. From example:  the following Main Effects could affect thick, From example:  the following Main Effects could affect thick, Green Grass Green Grass - - Type of grass seed Type of grass seed - - Type of soil Type of soil - - Amount of Fertilizer Amount of Fertilizer - - Amount of Water Amount of Water

7 Statistical Design of Experiments Statistical Design of Experiments Main Effect Descriptors Main Effect Descriptors Qualitative Qualitative -- -- category and/or order, category and/or order, alphanumeric alphanumeric Type of soil:  Clay, Top Soil Type of soil:  Clay, Top Soil Quantitative Quantitative -- -- numeric numeric Amount of water:  20 minutes of water Amount of water:  20 minutes of water per day; 60 minutes of water per day per day; 60 minutes of water per day

8 Statistical Design of Experiments Statistical Design of Experiments Main Effect Levels Main Effect Levels Level Level -- -- a given setting or amount of an Main Effect a given setting or amount of an Main Effect – – Set a Main Effect at two or more levels to determine if a Set a Main Effect at two or more levels to determine if a Response Variable improves at a given level Response Variable improves at a given level From the grass example: From the grass example: Type of Grass Seed Type of Grass Seed - - Level 1 = Red Fescue Level 1 = Red Fescue - - Level 2 = Perennial Level 2 = Perennial Rye Rye Amount of Fertilizer Amount of Fertilizer - - Level 1 = 2 bags per Level 1 = 2 bags per week week - - Level 2 = 1 bag per week Level 2 = 1 bag per week

9 Statistical Design of Experiments Statistical Design of Experiments A characteristic of the Design of A characteristic of the Design of Experiments method is the ability to Experiments method is the ability to test a combination of Main Effects at test a combination of Main Effects at different levels.  Therefore, Design of different levels.  Therefore, Design of Experiments allows the experimenter Experiments allows the experimenter to see how many Main Effects to see how many Main Effects affecting a product or process affecting a product or process interrelate. interrelate. Treatment Combination Treatment Combination

10 Statistical Design of Experiments Statistical Design of Experiments Treatment Treatment Combination Combination -- -- The The combination of Main Effects and combination of Main Effects and levels for each experimental trial levels for each experimental trial in a fractional or full factorial in a fractional or full factorial Design of Experiment. Design of Experiment. Treatment Combination Treatment Combination

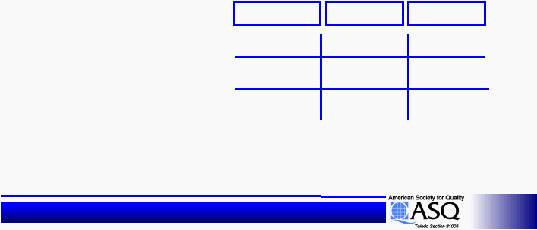11 Statistical Design of Experiments Statistical Design of Experiments Treatment Combination Treatment Combination Possible Possible Treatment Treatment Combination: Combination: Main Effect Main Effect Level 1 Level 1 Level 2 Level 2 Grass Seed Grass Seed Soil Type Soil Type Amount of Amount of Water Water Red Fescue Red Fescue Clay Clay 20 20 Min/Day Min/DayMin/Day Per. Rye Per. Rye Top Soil Top SoilTop Soil 60 60 Min/Day Min/Day Red Fescue, Red Fescue, Top Soil and Top Soil and 20 minutes 20 minutes water water Red Fescue Red Fescue 20 20 Min/Day Top Soil

12 Statistical Design of Experiments Statistical Design of Experiments Repeated Trial (Replication) Repeated Trial (Replication) Repeat a treatment combination 2 or Repeat a treatment combination 2 or more times. more times. A repeated trial is done to estimate A repeated trial is done to estimate the pure trial-to-trial estimate of the pure trial-to-trial estimate of error, so that the lack of fit may be error, so that the lack of fit may be judged. judged.

13 Statistical Design of Experiments Statistical Design of Experiments Statistical Design of Experiments Statistical Design of Experiments is Useful in Agriculture is Useful in Agriculture Agricultural Fields were Agricultural Fields were ideal for testing more ideal for testing more than one treatment than one treatment combination since land combination since land can be sectioned. can be sectioned.

14 Statistical Design of Experiments Statistical Design of Experiments Statistical Design of Experiments Statistical Design of Experiments is Useful in Agriculture is Useful in Agriculture Historically, Historically, DOE was used DOE was used in Agriculture as in Agriculture as well as well as Academia. Academia.

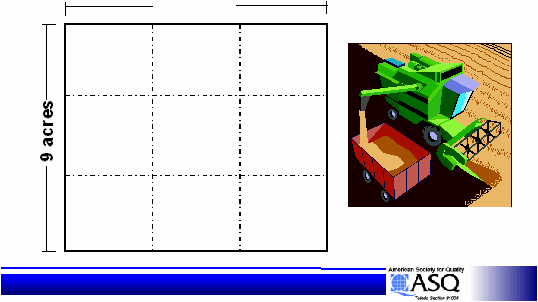15 Statistical Design of Experiments Statistical Design of Experiments Statistical Design of Experiments Statistical Design of Experiments is Useful in Agriculture is Useful in Agriculture 9 acres 9 acres Treatment Treatment Combination Combination #1 #1 Treatment Treatment Combination Combination # 2 # 2 Treatment Treatment Combination Combination # 3 # 3 Treatment Treatment Combination Combination # 4 # 4 Treatment Treatment Combination Combination # 5 # 5 Treatment Treatment Combination Combination # 6 # 6 Treatment Treatment Combination Combination # 7 # 7 Treatment Treatment Combination Combination # 8 # 8 Treatment Treatment Combination Combination # 9 # 9

16 Statistical Design of Experiments Statistical Design of Experiments Treatment Treatment combination combination results would results would be compared be compared for each section for each section of land to of land to determine the determine the best. best. Statistical Design of Experiments Statistical Design of Experiments is Useful in Agriculture is Useful in Agriculture

17 Statistical Design of Experiments Statistical Design of Experiments R. A. Fisher R. A. Fisher approach to approach to Design of Design of Experiments. Experiments. Statistical Design of Experiments Statistical Design of Experiments is Useful in Agriculture is Useful in Agriculture

18 Statistical Design of Experiments Statistical Design of Experiments For Example: For Example: A A corn farmer corn farmer desires to desires to increase corn increase corn yield.  The yield.  The farmer has farmer has sectioned his sectioned his land into 1 acre land into 1 acre plots ... plots ... Statistical Design of Experiments Statistical Design of Experiments is Useful in Agriculture is Useful in Agriculture

19 Statistical Design of Experiments Statistical Design of Experiments Corn Yield Improvement Corn Yield Improvement How can corn How can corn yield be yield be improved? improved?

20 Statistical Design of Experiments Statistical Design of Experiments Corn Yield Improvement Corn Yield Improvement What are the What are the Main Effects Main Effects which might help which might help to increase corn to increase corn yield? yield?

21 Statistical Design of Experiments Statistical Design of Experiments Corn Yield Improvement Corn Yield Improvement How many levels How many levels per Main Effect? per Main Effect?

22 Statistical Design of Experiments Statistical Design of Experiments Corn Yield Improvement Corn Yield Improvement At what levels At what levels should each should each Main Effect be Main Effect be tested?  tested?

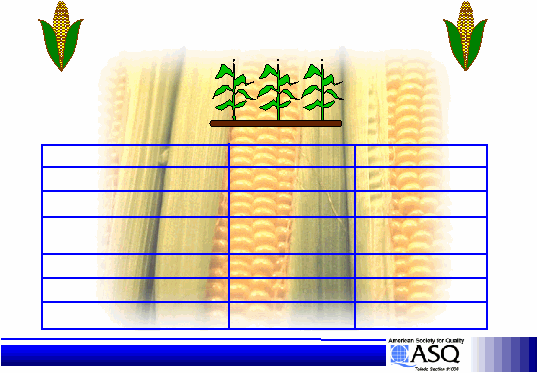23 Statistical Design of Experiments Statistical Design of Experiments Corn Yield Improvement Corn Yield Improvement Level 1 Level 1 Level 2 Level 2 Type of Seed Type of Seed Seed Population Seed Population Distance between Distance between corn plants corn plants A A 2 seeds per 2 seeds per Natural Natural B B 5 seeds per 5 seeds per 12 inches 12 inches Type of fertilizer Type of fertilizer A A B B Type of soil Type of soil 6 inches 6 inches Top soil Top soil Amount of water Amount of water Clay Clay Irrigation Irrigation Main Effects Main Effects

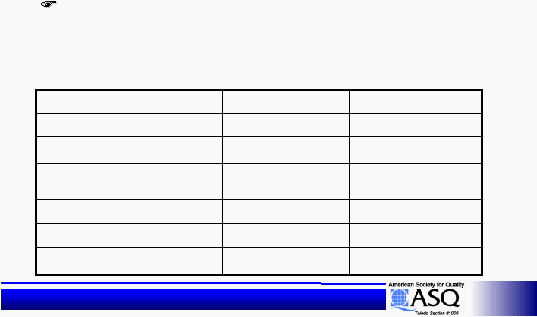24 Statistical Design of Experiments Statistical Design of Experiments Possible Treatment Combinations: Possible Treatment Combinations: Level 1 Level 1 Level 2 Level 2 Type of Seed Type of Seed Seed Population Seed Population Distance between corn Distance between corn plants plants A A 2 seeds per 2 seeds per Natural Natural B BB 5 seeds per 5 seeds per 12 inches 12 inches Type of fertilizer Type of fertilizer A AA B B Type of soil Type of soil 6 inches 6 inches Top soil Top soil Amount of water Amount of water Clay Clay Irrigation Irrigation Main Effects Main Effects Natural Natural BB 12 inches 12 inches B B 5 seeds per 5 seeds per Top soil Top soil A A A 6 inches 6 inches 5 seeds per 5 seeds per Top soil Top soil Irrigation Irrigation 2 seeds per 2 seeds per Clay Clay B 12 inches 12 inches A A Irrigation Irrigation Seed Type A, 5 seeds per, 6 inches Seed Type A, 5 seeds per, 6 inches between plants, fertilizer A, top soil, between plants, fertilizer A, top soil, irrigation irrigation

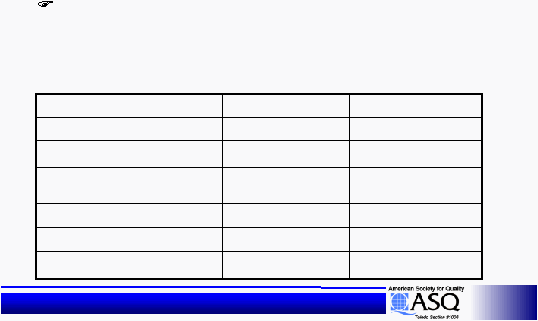25 Statistical Design of Experiments Statistical Design of Experiments Possible Treatment Combinations: Possible Treatment Combinations: Seed Type B, 5 seeds per, 12 inches Seed Type B, 5 seeds per, 12 inches between plants, fertilizer B, top soil, between plants, fertilizer B, top soil, natural water amount natural water amount Level 1 Level 1 Level 2 Level 2 Type of Seed Type of Seed Seed Population Seed Population Distance between corn Distance between corn plants plants A A 2 seeds per 2 seeds per Natural Natural B BB 5 seeds per 5 seeds per 12 inches 12 inches Type of fertilizer Type of fertilizer A AA B B Type of soil Type of soil 6 inches 6 inches Top soil Top soil Amount of water Amount of water Clay Clay Irrigation Irrigation Main Effects Main Effects Natural Natural BB 12 inches 12 inches B B 5 seeds per 5 seeds per Top soil Top soil A A A 6 inches 6 inches 5 seeds per 5 seeds per Top soil Top soil Irrigation Irrigation 2 seeds per 2 seeds per Clay Clay B 12 inches 12 inches A A Irrigation Irrigation

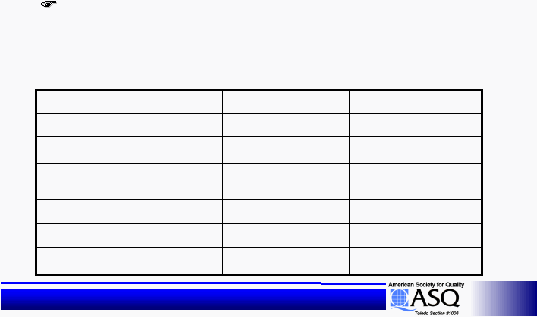26 Statistical Design of Experiments Statistical Design of Experiments Seed type B, 2 seeds per, 12 inches Seed type B, 2 seeds per, 12 inches between plants, fertilizer A, clay soil, between plants, fertilizer A, clay soil, irrigation, etc. irrigation, etc. Possible Treatment Combinations: Possible Treatment Combinations: Level 1 Level 1 Level 2 Level 2 Type of Seed Type of Seed Seed Population Seed Population Distance between corn Distance between corn plants plants A A 2 seeds per 2 seeds per Natural Natural B BB 5 seeds per 5 seeds per 12 inches 12 inches Type of fertilizer Type of fertilizer A AA B B Type of soil Type of soil 6 inches 6 inches Top soil Top soil Amount of water Amount of water Clay Clay Irrigation Irrigation Main Effects Main Effects Natural Natural BB 12 inches 12 inches B B 5 seeds per 5 seeds per Top soil Top soil A A A 6 inches 6 inches 5 seeds per 5 seeds per Top soil Top soil Irrigation Irrigation 2 seeds per 2 seeds per Clay Clay B 12 inches 12 inches A A Irrigation Irrigation

27 Statistical Design of Experiments Statistical Design of Experiments Possible Treatment Combinations: Possible Treatment Combinations: Each of the Each of the sections of land will sections of land will receive a different receive a different treatment treatment combination. combination.

28 Statistical Design of Experiments Statistical Design of Experiments As the corn is As the corn is harvested, the harvested, the corn yield for each corn yield for each of the sections of the sections will be will be determined. determined. Possible Treatment Combinations: Possible Treatment Combinations:

29 Statistical Design of Experiments Statistical Design of Experiments The section The section (treatment (treatment combination) combination) which yields the which yields the most corn .... most corn .... Possible Treatment Combinations: Possible Treatment Combinations:

30 Statistical Design of Experiments Statistical Design of Experiments Applicable to: Applicable to: New Designs / New Processes New Designs / New Processes Existing Designs / Existing Existing Designs / Existing Processes Processes Statistical Design of Experiments Statistical Design of Experiments

31 Statistical Design of Experiments Statistical Design of Experiments Drivers of DOE Drivers of DOE Customer Led Quality Customer Led Quality Robust Designs / Processes Robust Designs / Processes Loss to Society Loss to Society Statistical Design of Experiments Statistical Design of Experiments

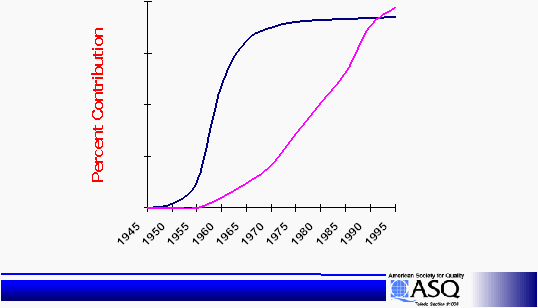
32 Statistical Design of Experiments Statistical Design of Experiments Foreign Competition Foreign Competition -- -- Success Success stories from Japan on how stories from Japan on how application of Statistical Methods application of Statistical Methods helped get the cost out and helped get the cost out and improve quality. improve quality. Statistical Design of Experiments Statistical Design of Experiments

33 Statistical Design of Experiments Statistical Design of Experiments Japanese Quality Evolution Japanese Quality Evolution 0 25 50 75 100 Quality Quality Due to Inspection Due to Inspection Quality Due to Quality Due to Process Process Control Control Quality Due to Quality Due to Design of Design of Experiments Experiments

34 Statistical Design of Experiments Statistical Design of Experiments Design of Experiments - Design of Experiments - Steps Steps 1. Recognition of and Statement of the Problem 2. Selection of the response variable 3. Choice of Factors and Levels 4. Choice of experimental design 5. Performing the experiment 6. Data analysis 7. Proof test of Optimal Treatment Combination 8. Conclusions and recommendations 9. Write It Up !! 1. Recognition of and Statement of the Problem 2. Selection of the response variable 3. Choice of Factors and Levels 4. Choice of experimental design 5. Performing the experiment 6. Data analysis 7. Proof test of Optimal Treatment Combination 8. Conclusions and recommendations 9. Write It Up !!

35 Statistical Design of Experiments Statistical Design of Experiments Background: Background: CMEP is having a problem CMEP is having a problem with the variability of B-160 rotary fuel pump with the variability of B-160 rotary fuel pump timing.  A designed experiment has been timing.  A designed experiment has been used to determine what main effects affect used to determine what main effects affect fuel pump timing.  During the brainstorming fuel pump timing.  During the brainstorming session, many main effects are discussed, session, many main effects are discussed, and listed below  are the 7 main effects and listed below  are the 7 main effects thought to have the best possibility of thought to have the best possibility of affecting fuel pump timing. affecting fuel pump timing. Exercise 1 - Exercise 1 - Case Study Case Study

36 Statistical Design of Experiments Statistical Design of Experiments Objective: Objective: To determine which of To determine which of the selected Main Effects affect the selected Main Effects affect Fuel Pump Timing. Fuel Pump Timing. Exercise 1 - Exercise 1 - Case Study Case Study

37 Statistical Design of Experiments Statistical Design of Experiments Main Effect Main Effect A)  Input air pressure A)  Input air pressure B)  Fuel pump mounting B)  Fuel pump mounting C)  TDC set point C)  TDC set point D)  Fuel pump mtg D)  Fuel pump mtg nut nut torque seq. torque seq. E)  Fuel pump gear torque E)  Fuel pump gear torque F)  Backlash tool F)  Backlash tool G)  Associate G)  Associate Exercise 1 - Exercise 1 - Case Study Case Study LEVEL 1 LEVEL 1 100 psi 100 psi Snug Snug 0.75 deg. 0.75 deg. Top first Top first Two step Two step METC METC Bob Bob LEVEL 2 LEVEL 2 80 psi 80 psi Loose Loose 0 deg. 0 deg. Bottom first Bottom first One step One step Spring design Spring design Jim Jim

38 Statistical Design of Experiments Statistical Design of Experiments Background: Background: CMEP is having a problem CMEP is having a problem with the variability of B-160 rotary fuel pump with the variability of B-160 rotary fuel pump timing.  A designed experiment has been timing.  A designed experiment has been used to determine what main effects affect used to determine what main effects affect fuel pump timing.  During the brainstorming fuel pump timing.  During the brainstorming session, many main effects are discussed, session, many main effects are discussed, and listed below  are the 7 main effects and listed below  are the 7 main effects thought to have the best possibility of thought to have the best possibility of affecting fuel pump timing. affecting fuel pump timing. Exercise 1 - Exercise 1 - Case Study Case Study

39 Statistical Design of Experiments Statistical Design of Experiments Exercise 1 Cont’d. Exercise 1 Cont’d. 1. 1. What is the response variable?  What is What is the response variable?  What is another name for the response variable? another name for the response variable? 2. 2. How many Main Effects are there?  How many Main Effects are there?  Identify 3 Main Effects. Identify 3 Main Effects. 3. 3. Identify the levels for the Main Effects Identify the levels for the Main Effects that you chose in question #2. that you chose in question #2. 4. 4. Are all the Main Effects in this Are all the Main Effects in this experiment Qualitative, Quantitative, or experiment Qualitative, Quantitative, or both?  Identify examples of each. both?  Identify examples of each.

40 Statistical Design of Experiments Statistical Design of Experiments Exercise 1 Cont’d. Exercise 1 Cont’d. 1. 1. What is the response variable?  What is What is the response variable?  What is What is another name for the response variable? another name for the response variable? Fuel Pump Timing Fuel Pump Timing Answer: Answer: Output Variable, Output Variable, Dependant Variable Dependant Variable

41 Statistical Design of Experiments Statistical Design of Experiments Exercise 1 Cont’d. Exercise 1 Cont’d. 2. 2. How many Main Effects are there? How many Main Effects are there? Identify 3 Main Effects. Identify 3 Main Effects. Seven Seven Answer: Answer: Input Air Pressure, Input Air Pressure, Fuel Pump Mounting Unit Fuel Pump Mounting Unit TDC Set Point TDC Set Point Fuel Pump Mtg Fuel Pump Mtg Mtg nut Torque Seq. nut Torque Seq. Fuel Pump Gear Torque Fuel Pump Gear Torque Backlash Tool Backlash Tool Associate Associate

42 Statistical Design of Experiments Statistical Design of Experiments Exercise 1 Cont’d. Exercise 1 Cont’d. 3. 3. Identify the levels for the Main Effects Identify the levels for the Main Effects that you chose in question #2. that you chose in question #2. Answer: Answer: Main Effect Main Effect LEVEL 1 LEVEL 1 LEVEL 2 LEVEL 2 A)  Input air pressure A)  Input air pressure 100 psi 100 psi psi 80 psi 80 psi psi B)  Fuel pump mounting nut B)  Fuel pump mounting nut Snug Snug Loose                          Loose                          C)  TDC set point C)  TDC set point 0.75 deg. before 0.75 deg. before TDC - TDC - - top dead     top dead     ctr ctr (0 deg)        (0 deg)        D)  Fuel pump mtg D)  Fuel pump mtg mtg nut torque seq. nut torque seq. Top first Top first Bottom first               Bottom first               E)  Fuel pump gear torque E)  Fuel pump gear torque Two step Two step One step                     One step                     F)  Backlash tool F)  Backlash tool METC METC Spring design           Spring design           G)  Associate G)  Associate Bob Bob Jim      Jim

43 Statistical Design of Experiments Statistical Design of Experiments Exercise 1 Cont’d. Exercise 1 Cont’d. 4. 4. Are all the Main Effects in this experiment Are all the Main Effects in this experiment Qualitative, Quantitative, or both?  Qualitative, Quantitative, or both?  Identify examples of each. Identify examples of each. Both ! Both ! Answer: Answer: Quantitative = A, C Quantitative = A, C Qualitative = B, D, E, F, G Qualitative = B, D, E, F, G

44 Statistical Design of Experiments Statistical Design of Experiments Definitions - Definitions - Reference Reference

45 Statistical Design of Experiments Statistical Design of Experiments Experiment Experiment - - An operation under controlled conditions An operation under controlled conditions (usually off line) to determine an unknown (usually off line) to determine an unknown response variable effect response variable effect Main Effect Main Effect - - The Variables which are manipulated or The Variables which are manipulated or controlled during the experiment. controlled during the experiment. Response Variable Response Variable - - The Variable that is observed                              The Variable that is observed                              or measured. or measured. Definitions - Definitions - Reference Reference

46 Statistical Design of Experiments Statistical Design of Experiments Levels – – The values of the Main Effects in the experiment The values of the Main Effects in the experiment • • Example:  90 degrees = level 1 fuel temp. Example:  90 degrees = level 1 fuel temp. 110 degrees = level 2 fuel temp. 110 degrees = level 2 fuel temp. Effect – – The change in the response variable caused by an The change in the response variable caused by an Main Effect moving from one level to another Main Effect moving from one level to another Treatment Combination – – The specific combinations of levels of all the input         The specific combinations of levels of all the input         factors factors Definitions - Definitions - Reference Reference

47 Statistical Design of Experiments Statistical Design of Experiments End End of of Unit One Unit One